Novel approach peak tracking method for FBG: Gaussian polynomial technique

Abstract

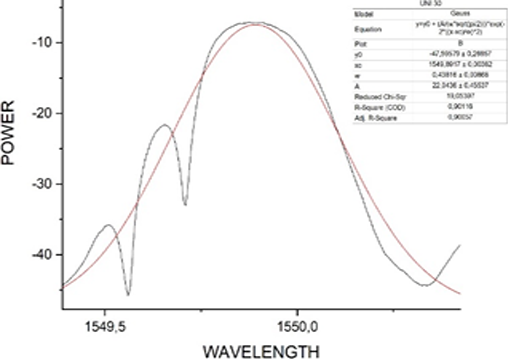
This paper presents a novel approach for tracking the peaks in the FBG spectrum using the Gaussian polynomial method. The proposed algorithm involves preprocessing the FBG signal, detecting the peaks, and fitting the peaks with a Gaussian function. The performance of the algorithm is evaluated using both simulated and experimental FBG spectra. This method involves fitting a Gaussian function to the peak of interest and using the fitted parameters to estimate peak height, width, and location. The method is highly accurate and precise and can provide detailed information about peak shape and position, making it effective for tracking complex or overlapping peaks. However, the method can be computationally intensive and may require careful selection of initial parameters to ensure accurate results. Despite these limitations, the Gaussian polynomial method is a powerful tool for peak tracking and analysis in various application.